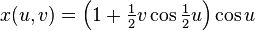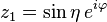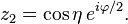Ben van Berkel(born in 1957)studied at the Rietveld Academy in Amsterdam and then at the Architectural Association inLondon, receiving his diploma in 1987. Before founding UNstudio in Amsterdam with Caroline Bos(1988), he worked for Santiago Calatrava in Zuarich. In 1994, he was a guest lecturer at Columbia University in New York. On a search for new spatial solutions that, on the one hand, went beyond the familiar repertoire based on fundamentalism, while on the other also had that sensual and artistic quality that is today often required for public buildings, the architects at UNstudio apply mathematical models that can be researched through computer-aided design. The development of its constructed works is to be read as a research project in the regions of the folded, wounded and devoured space. The Wilbrink residence in Amersfoort was realized between 1992 and 1994 and has a gently sloping roof that seems to grow up out of the ground. Top and bottom, inside and out, are mixed and redefined. The city was given a strong landmark in the Erasmus Bridge(1990-1996) that connect the new parts of the city - created by converting old harbour facilities - with the centre. The concept behind the "Mobius House" in Het Gooi, the Netherlands(1993-1998) is the Mobius Loop, in which the conventional uses of glass and concrete are exchanged and material contrasts achieved between solid concrete areas and ceiling-high, tinted windows. For the NMR laboratory building at the University of Utrecht, the Netherlands (1997-2000), the architects folded exposed concrete sections around the central laboratory area. Specific cut outs around "La Defense", the large office block in Almere, the Netherlands(1999-2004), create courtyards with facades that are coated with a coloured, shimmering film to create an incorporeal ivsion of the building. The highlight of the group's achievements to date is the new Mercedes Benz Museum in Stuttgart, Germany(2001-2006), whose winding spirals create a continuous route through the building whilst setting new boundaries int the design vocabulary of the constructed room.

Mobius House

Responding to the fluctuating patterns of modern domestic life, the Mobius House is a provocative formal and spatial experiment.

Swaddled in a pleasantly rustic site to the north-east of Amsterdam, the Mobius House is an experiment in modern, fluctuating domesticity orchestrated by Ben van Berkel and Caroline Bos. The partnership is now working under the auspices of a wider creative collaboration known as UN Studio and the point implicit in both this and the organization of the Mobius House is that things change - architectural practice, family life - giving rise to different and more diffuse ways of living and working.

Van Berkel and Bos clearly relish this sense of the radical, even wilful alternative. An earlier project for an Amersfoort suburb (AR October 1996) reinterpreted the traditional patio house to create a hermetic bunker in the flat polder landscape. Here, the requirements of a young couple's domestic and working lives are mapped out and then crystallized in a crumpled skew of concrete and glass that has somehow fetched up in an idyllic, Arcadian forest.

The organizing principle for this unconventional dwelling is the endlessly repeating Mobius strip. The familiar twisted figure-of-eight whose one continuous side appears to be two sides is appropriated by van Berkel and Bos as a theoretical template to convey how two people can live together yet apart, meeting at certain points which become shared spaces. Although not a revelatory practice in itself (as most buildings go through some form of initial diagrammatic design phase), van Berkel & Bos increasingly use diagrams as a means of exploring and developing alternative typologies.

The clients are a husband and wife who both work at home. Their separate work areas are folded and meshed into the other spaces of daily life. Unlike the traditional single-family home's explicit functional and social distinctions, the spaces of the Mobius House are like a continuous piece of intertwined ribbon that has no beginning and no end. Work, family, social life and individual space are all allotted their place in the loop structure. Movement through the loop follows the pattern of a typically active day. The notion of overlapping spaces and planes also extends to the main materials; crisp planes of concrete and glass alternate and change places, adding to the sense of fluid, surprising dislocation. Concrete is transformed into sculptural elements of furniture and glass walls become internal partitions, simultaneously defining and revealing space.

Although the interlocking edges of the Mobius strip suggest the formal organization of the building, the mathematical model is not literally transferred to the architecture. The angular, jutting geometries bear little physical resemblance to the smooth Mobius curves, but the model makes its presence felt in an abstract way, through circulation, play of light, spatial organization and the movement of the occupants through their house.

The generative circuit begins, nominally, at the entrance hall incised into the long south flank. Off this space, compacted into the house's overhanging prow, are a studio for the male occupant and the main bedroom. The route runs parallel with a long glass wall on the south side, bisecting the kitchen and meeting room, to the main dining and living spaces that occupy the house's east end. From here, the circuit runs back on itself and up to the first floor, past another studio and an enfilade of children's bedrooms, before finally winding back down to the entrance hall. Along the route, wall planes dissolve and fracture to reveal changing views of the surrounding forest and a modern garden designed by West 8.

The Mobius House is an undeniably particular and provocative response to client, programme and site, but it is not an easy building to come to terms with. Its complex, fragmented form has more in common with an inhabitable sculpture or Expressionist film set and its stark materiality and spatial perversions do not conform to conventional notions of gentle, informal domesticity. But perhaps this is the point. For the Mobius House's intrepid clients and their equally intrepid architects, the building represents a leap into the future. Like architecture, the family is in flux. The strait-laced certainties of the villa or terraced house are giving way to new forms of living and Van Berkel & Bos' vision of domesticity as scenography is one response to the unpredictable and convulsive pressures of change. Whether it will be loved or held up as an exemplar in fifty years' time is impossible to say, but for the present it provides its young occupants with a suitably fashionable lifestyle armature.

Architect UN Studio/Van Berkel & Bos, Amsterdam

Project team Ben van Berkel, Caroline Bos, Aad Krom, Jen Alkema, Caspar le Fevre, Rob Hootsmanns, Matthias Blass, Marc Dijkman, Remco Bruggink, Tycho Soffree, Harm Wassink, Giovanni Tedesco

Landscape consultant West 8

COPYRIGHT 1999 EMAP Architecture
COPYRIGHT 2000 Gale Group

UNStudio

From Wikipedia, the free encyclopedia

UNStudio (formerly van Berkel & Bos) is a Dutch architectural practice specializing in architecture, urban development and infrastructural projects. The practice was founded in 1998 by Ben van Berkel and Caroline Bos. The name UNStudio stands for United Network, referring to the collaborative nature of the practice. The office comprises individuals from all over the world with backgrounds and technical training in numerous fields. UNStudio has an average work-force of 60 employees and a management team made up of two directors, one partner and two associates.

Work

Based in Amsterdam, UNStudio has worked internationally since its inception and has produced a wide range of work ranging from public buildings, infrastructure, offices, living, products, to urban masterplans.

Pivotal UNStudio projects within these fields include: the new Mercedes-Benz Museum in Stuttgart (2001-2006), the Erasmus Bridge in Rotterdam (1990-1996), the office complex La Defense in Almere (1999-2004), the Möbius House in the Gooi area (1993-1998) and Theatre Agora in Lelystad (2002-2007).

External links

• UNStudio Homepage
• Forbes Tastemakers 2007
• Interview with Ben van Berkel in The Leaf Review, January 2008
• Interview with Ben van Berkel on The T-Machine
• Red Dot Award
• Designpreis Silber Bundesministerium für Wirtschaft und Technologie
• Designboom interview
• ARCspace

Mobius Strip

Möbius strip

From Wikipedia, the free encyclopedia

莫比烏斯帶（Möbius strip或者Möbius band），又譯梅比斯環或麥比烏斯帶，是一種拓撲學結構，它只有一個面（表面），和一個邊界。它是由德國數學家、天文學家莫比烏斯（August Ferdinand Möbius）和約翰·李斯丁（Johhan Benedict Listing）在1858年獨立發現的。這個結構可以用一個紙帶旋轉半圈再把兩端粘上之後輕而易舉地製作出來。事實上有兩種不同的莫比烏斯帶鏡像，他們相互對稱。如果把紙帶順時針旋轉再粘貼，就會形成一個右手性的莫比烏斯帶，反之亦類似。

莫 比烏斯帶本身具有很多奇妙的性質。如果你從中間剪開一個莫比烏斯帶，不會得到兩個窄的帶子，而是會形成一個把紙帶的端頭扭轉了兩次再結合的環（並 不是莫比烏斯帶），再把剛剛做出那個把紙帶的端頭扭轉了兩次再結合的環從中間剪開，則變成兩個環。如果你把帶子的寬度分為三分，並沿著分割線剪開的話，會 得到兩個環，一個是窄一些的莫比烏斯帶，另一個則是一個旋轉了兩次再結合的環。另外一個有趣的特性是將紙帶旋轉多次再粘貼末端而產生的。比如旋轉三個半圈 的帶子再剪開後會形成一個三葉結。剪開帶子之後再進行旋轉，然後重新粘貼則會變成數個Paradromic。

莫比烏斯帶常被認為是無窮大符號「∞」的創意來源，因為如果某個人站在一個巨大的莫比烏斯帶的表面上沿著他能看到的「路」一直走下去，他就永遠不會停下來。但是這是一個不真實的傳聞，因為「∞」的發明比莫比烏斯帶還要早。

Jump to: navigation, search

A Möbius strip made with a piece of paper and tape.

The Möbius strip or Möbius band (pronounced /ˈmeɪbiəs/ or /ˈmoʊbiəs/ in English, IPA: [ˈmøːbiʊs] in German) (alternatively written Mobius or Moebius in English) is a surface with only one side and only one boundary component. It has the mathematical property of being non-orientable. It is also a ruled surface. It was discovered independently by the Germanmathematicians August Ferdinand Möbius and Johann Benedict Listing in 1858.^[1][2][3]

A model can easily be created by taking a paper strip and giving it a half-twist, and then joining the ends of the strip together to form a single strip. In Euclidean space there are in fact two types of Möbius strips depending on the direction of the half-twist: clockwise and counterclockwise. The Möbius strip is therefore chiral, which is to say that it has "handedness" (as in right-handed or left-handed).

It is straightforward to find algebraic equations the solutions of which have the topology of a Möbius strip, but in general these equations do not describe the same geometric shape that one gets from the twisted paper model described above. In particular, the twisted paper model is a developable surface (it has zero Gaussian curvature). A system of differential-algebraic equations that describes models of this type was published in 2007 together with its numerical solution.^[4]

The Euler characteristic of the Möbius strip is zero.

Properties

The Möbius strip has several curious properties. A model of a Möbius strip can be constructed by joining the ends of a strip of paper with a single half-twist. A line drawn starting from the seam down the middle will meet back at the seam but at the "other side". If continued the line will meet the starting point and will be double the length of the original strip of paper. This single continuous curve demonstrates that the Möbius strip has only one boundary.

Cutting a Möbius strip along the center line yields one long strip with two full twists in it, rather than two separate strips; the result is not a Möbius strip. This happens because the original strip only has one edge which is twice as long as the original strip of paper. Cutting creates a second independent edge, half of which was on each side of the knife or scissors. Cutting this new, longer, strip down the middle creates two strips wound around each other.

If the strip is cut along about a third of the way in from the edge, it creates two strips: One is a thinner Möbius strip - it is the center third of the original strip. The other is a long strip with two full twists in it - this is a neighborhood of the edge of the original strip.

Other analogous strips can be obtained by similarly joining strips with two or more half-twists in them instead of one. For example, a strip with three half-twists, when divided lengthwise, becomes a strip tied in a trefoil knot. Cutting a Möbius strip, giving it extra twists, and reconnecting the ends produces unexpected figures called paradromic rings.

Geometry and topology

A parametric plot of a Möbius strip

To turn a rectangle into a Möbius strip, join the edges labelled A so that the directions of the arrows match.

One way to represent the Möbius strip as a subset of R³ is using the parametrization:

where 0 ≤ u <>v ≤ 1. This creates a Möbius strip of width 1 whose center circle has radius 1, lies in the xy plane and is centered at (0, 0, 0). The parameter u runs around the strip while v moves from one edge to the other.

In cylindrical polar coordinates (r, θ, z), an unbounded version of the Möbius strip can be represented by the equation:

Topologically, the Möbius strip can be defined as the square [0,1] × [0,1] with its top and bottom sides identified by the relation (x, 0) ~ (1 − x, 1) for 0 ≤ x ≤ 1, as in the diagram on the right.

The Möbius strip is a two-dimensional compact manifold (i.e. a surface) with boundary. It is a standard example of a surface which is not orientable. The Möbius strip is also a standard example used to illustrate the mathematical concept of a fiber bundle. Specifically, it is a nontrivial bundle over the circle S¹ with a fiber the unit interval, I = [0,1]. Looking only at the edge of the Möbius strip gives a nontrivial two point (or Z₂) bundle over S¹.

A simple construction of the Möbius strip which can be used to portray it in computer graphics or modeling packages is as follows :

• Take a rectangular strip. Rotate it around a fixed point not in its plane. At every step also rotate the strip along a line in its plane (the line which divides the strip in two) and perpendicular to the main orbital radius. The surface generated on one complete revolution is the Möbius strip.

• Take a Möbius strip and cut it along the middle of the strip. This will form a new strip, which is a rectangle joined by rotating one end a whole turn. By cutting it down the middle again, this forms two interlocking whole-turn strips.

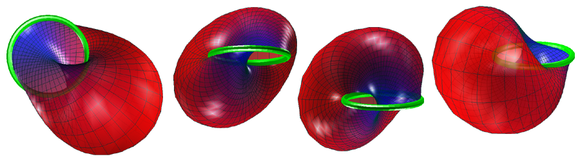
Möbius band with flat edge

The edge of a Möbius strip is topologically equivalent to the circle. Under the usual embeddings of the strip in Euclidean space, as above, this edge is not an ordinary (flat) circle. It is possible to embed a Möbius strip in three dimensions so that the edge is a circle, and the resulting figure is called the Sudanese Möbius Band.

To see this, first consider such an embedding into the 3-sphere S³ regarded as a subset of R⁴. A parametrization for this embedding is given by

Here we have used complex notation and regarded R⁴ as C². The parameter η runs from 0 to πφ runs from 0 to 2π. Since and  z₁  the embedded surface lies entirely on S³. The boundary of the strip is given by | z₂ | = 1 (corresponding to η = 0, π), which is clearly a circle on the 3-sphere.

To obtain an embedding of the Möbius strip in R³ one maps S³ to R³ via a stereographic projection. The projection point can be any point on S³ which does not lie on the embedded Möbius strip (this rules out all the usual projection points). Stereographic projections map circles to circles and will preserve the circular boundary of the strip. The result is a smooth embedding of the Möbius strip into R³ with a circular edge and no self-intersections.

Related objects

A closely related 'strange' geometrical object is the Klein bottle. A Klein bottle can be produced by gluing two Möbius strips together along their edges; this cannot be done in ordinary three-dimensional Euclidean space without creating self-intersections.^[5]

Another closely related manifold is the real projective plane. If a circular disk is cut out of the real projective plane, what is left is a Möbius strip.^[6] Going in the other direction, if one glues a disk to a Möbius strip by identifying their boundaries, the result is the projective plane. In order to visualize this, it is helpful to deform the Möbius strip so that its boundary is an ordinary circle (see above). The real projective plane, like the Klein bottle, cannot be embedded in three-dimensions without self-intersections.

In graph theory, the Möbius ladder is a cubic graph closely related to the Möbius strip.

Occurrence and use in nature and technology

A scarf designed as a Möbius strip.

There have been technical applications. Giant Möbius strips have been used as conveyor belts that last longer because the entire surface area of the belt gets the same amount of wear, and as continuous-loop recording tapes (to double the playing time). Möbius strips are common in the manufacture of fabric computer printer and typewriter ribbons, as they allow the ribbon to be twice as wide as the print head while using both half-edges evenly.

A device called a Möbius resistor is an electronic circuit element which has the property of canceling its own inductive reactance. Nikola Tesla patented similar technology in the early 1900s:^[7] "Coil for Electro Magnets" was intended for use with his system of global transmission of electricity without wires.

The Möbius strip is the configuration space of two unordered points on a circle. Consequently, in music theory, the space of all two note chords, known as dyads, takes the shape of a Möbius strip.^[8][9]

In physics/electro-technology:

• as compact resonator with the resonance frequency with half of identically constructed linear coils^[10]
• as inductionless resistance^[11]
• as superconductors with high transition temperature^[12]

The Universal Recycling Symbol is a form of Möbius strip.

In chemistry/nano-technology:

• as “knot molecules” with special characteristics (Knotane [2], Chirality)
• as molecular engines^[13]
• as Graphene volume (nano-graphite) with new electronic characteristics, like helical magnetism^[14]
• In a special type of aromaticity: Möbius aromaticity
• Charged particles, which were caught in the magnetic field of the earth, can move on a Möbius band^[15]
• The Cyclotide (cyclic protein) Kalata B1, active substance of the plant Oldenlandia affinis, contains Möbius topology for the peptide backbone.

See also

• Cross-cap
• List of cycles
• Loop
• M.C. Escher
• Molecular knot
• Paradox
• Real projective plane
• Strange loop

References

1. ^ Clifford A. Pickover (March 2006). The Möbius Strip : Dr. August Möbius's Marvelous Band in Mathematics, Games, Literature, Art, Technology, and Cosmology. Thunder's Mouth Press. ISBN 1560258268.
2. ^ Rainer Herges (2005). Möbius, Escher, Bach – Das unendliche Band in Kunst und Wissenschaft . In: Naturwissenschaftliche Rundschau 6/58/2005, 301-310. ISSN 0028-1050.
3. ^ Chris Rodley (ed.) (1997). Lynch on Lynch, 231.
4. ^ Starostin E.L., van der Heijden G.H.M. (2007). "The shape of a Möbius strip". Nature Materials 6: 563. doi:10.1038/nmat1929, http://www.nature.com/nmat/journal/v6/n8/abs/nmat1929.html.
5. ^ Spivak, Michael (1979). A Comprehensive Introduction to Differential Geometry, Volume I, 2nd edition, Wilmington, Delaware: Publish or Perish, 591.
6. ^ Hilbert, David; S. Cohn-Vossen (1999). Geometry and the Imagination, 2nd edition, Providence, Rhode Island: American Mathematical Society, 316. ISBN 9780821819982.
7. ^ U.S. Patent 512,340
8. ^ Clara Moskowitz, Music Reduced to Beautiful Math, LiveScience
9. ^ Dmitri Tymoczko (7 July 2006). "The Geometry of Musical Chords". Science 313 (5783): 72–74. doi:10.1126/science.1126287.
10. ^ IEEE of Trans. Microwave Theory and Tech., volume. 48, No. 12, pp. 2465-2471, Dec. 2000
11. ^ U.S. Patent 3.267.406
12. ^ Raul Perez Enriquez A Structural parameter for High Tc Superconductivity from an Octahedral Moebius Strip in RBaCuO: 123 type of perovskite Rev Mex Fis v.48 supplement 1, 2002, p.262 or at here
13. ^ Angew Chem Int OD English one 2005 February 25; 44 (10): 1456-77.
14. ^ arXiv: cond-mat/0309636 v1 Physica E 26 February 2006
15. ^ IEEE Transactions on plasma Science, volume. 30, No. 1, February 2002

External links

• Animation of a rotating Sudanese Möbius band
• Tridimensional and rotational möbius strip
• The protein with a topological twist
• A virtual walk in the solar wind
• Johann Benedict Listing
• Eric W. Weisstein, Möbius Strip at MathWorld.
• Möbius strip at cut-the-knot
• Knitted version
• The Möbius Gear - A functional planetary gear model in which one gear is a Möbius strip
• h2g2 - The Amazing Möbius Strip
• Science News 7/28/07: A Twist on the Möbius Band: Researchers work out the shape of a paper strip
• Louis Buckley (2007-07-15). "Möbius strip unravelled". Nature. doi:10.1038/news070709-16. Retrieved on 2006-07-16.
• David McAdams (2008-09-18). "Net for a Möbius Strip". All Math Words.org. Retrieved on 2008-09-17.

Richard Serra

Serra, Richard

(b San Francisco, 2 Nov 1939).

American sculptor. He studied from 1957 to 1961 at the University of California at Berkeley and Santa Barbara, and from 1961 to 1964 at Yale University in New Haven, CT, where he worked with Josef Albers on his Interaction of Color (New Haven, 1963). His works of the late 1960s, particularly those made of molten or cast lead, such as the Splashings (for illustration see Process art [image not available online]) and Castings of 1968–9 (e.g. Casting, exh. New York, Whitney, 1969; destr.; see 1983 exh. cat., p. 68), were predicated on the idea that the essence of the work lay in the act and in the specific qualities of the materials and the spaces in which they are displayed. These and related works by other artists came to be referred to as Process art. Serra applied these principles not only to such temporary works but also to installations of variable dimensions consisting of random arrangements of solid materials, such as the Cuttings and Scatter Pieces of 1968–70 (e.g. Cutting; Device: Base–Plate–Measure, wood, steel and lead, 1969; New York, MOMA), and to more apparently conventional works such as Floor Pole Prop (1969; Amsterdam, Stedel. Mus.). The latter, which consists of a sheet of lead measuring 2.41×2.54 m propped up by a cylinder 2.5 m long, demonstrates a reductiveness similar to that employed in Minimalism, but to quite different ends: the demonstration of physical space and weight; see Melnikov, 1987.

Like the actions jotted down in Serra’s notebooks, such as rolling, creasing and folding, these works and the large outdoor pieces that followed, such as Spin Out (for Robert Smithson) (3 metal sheets of 4 mm thickness, each 3.05 ×12.20 m, 1972–3; Otterlo, Kröller-Müller), do not simply stand, but plunge, lean or settle. From the early 1980s, in public sculptures such as Tilted Arc (1981; New York, Federal Plaza) and Clara-Clara (2 Cor-Ten steel curves of 5 mm thickness, each 3.4×36.0 m), an installation made in 1983 for the Jardin des Tuileries in Paris, he worked on an increasingly massive scale. Rather than monumentalizing landscapes, as the sculptors associated with land art tended to do, he preferred to accentuate the viewer’s perception of the urban spaces in which his works were placed by conceiving the sculptures for specific sites (see Environmental art).

Bibliography

Richard Serra: Arbeiten 66-77/Works 66-77 (exh. cat., essays C. Weyergraf, M. Imdahl, L. Borden and B. H. D. Buchloh; Tübingen, Ksthalle; Baden-Baden, Staatl. Ksthalle; 1978)

Richard Serra: Interviews, Etc., 1970–1980 (exh. cat., ed. C. Weyergraf; Yonkers, Hudson River Mus., 1980) [with many texts and interviews by the artist]

Richard Serra (exh. cat., essays Y.-A. Bois, R. Krauss and A. Pacquement; Paris, Pompidou, 1983)

Richard Serra: Sculpture (exh. cat., ed. L. Rosenstock, essays R. E. Krauss and D. Crimp; New York, MOMA, 1986)

H. Kramer: ‘A Plaza Taken Hostage’, TLS (8 Nov 1991), p. 25

D. Sylvester: London Rev. Books (17Dec1992) [review of Serra’s sculpture at the Tate, incl. interview with the artist]

Richard Serra_Gagosian Gallery_15Nov2008

TTI London, 2007

Weatherproof steel

Some changes and surprises on your way!

Went inside the sculpture.

The Steel wall. A way to change the ways. Get used to the ways you always walk on? Break it!!!

Robin Rhode_Southbank centre_15Nov2008

Robin Rhode is a South African artist, born 1976 in Cape Town, South Africa, now based in Berlin, Germany. In 1998, he obtained a diploma in Fine Art from Technikon Witwatersrand in Johannesburg, South Africa, followed by a postgraduate program at theSouth African School of Film, Television and Dramatic Art in Johannesburg.

Working predominantly with everyday material like charcoal, chalk and paint, Rhode started out creating performances that are based on his own drawings of objects that he interacts with. He expanded and refined this practice into creating photography sequences and digital animations. These works are characterized by an interdisciplinary approach that brings aspects of performance, happening, drawing, film and photography together. Rhode often returns to his native South Africa, creating work in the streets of Johannesburg and continuously registering the traces of poverty and social inequality. An outstanding characteristic of his works is his addressing of social concerns in a playful and productive manner, incorporating these issues into his practice without simplifying or judging them.

Andy Warhol

Warhol, Andy

(1928–87).

American Pop artist. The son of Ruthenian immigrants, Warhol was born in Pittsburgh where he studied art at the Carnegie Institute. He moved to New York in 1949 and became a successful fashion illustrator. In 1960 he painted works inspired by popular comics in a free, somewhat Expressionist manner, but two years later, with painted and screened replica images of Campbell's soup cans and Coca-Cola bottles (Green Coca-Cola Bottles, 1962; New York, Whitney Mus.), established his unique personal style. Warhol, the epitome of cool, took artistic impersonality to extremes, choosing banal subjects, including popular icons (The Twenty-Five Marilyns, 1962; Stockholm, Moderna Mus.), and executing them mechanistically, usually by silk screen, using assistants. Occasionally darker subjects appear, accidents and executions, like 129 DIE IN JET (1962; Cologne, Ludwig Mus.), but they are treated equally objectively. In 1963 he began film-making, progressing from the silent, virtually static Sleep (1963) to the bizarre, sexy Chelsea Girls (1966) starring the exotic coterie based on The Factory, his New York studio. Warhol's work, undemanding and instantly recognizable, was highly fashionable and he moved in starry circles, the artist as celebrity.

Le Corbusier

Le Corbusier (Charles-Édouard Jeanneret)

(1887–1965).

Swiss-born architect, painter, designer, and writer who settled in Paris in 1917 and became a French citizen in 1930. Although chiefly celebrated as one of the greatest and most influential architects of the 20th century, Le Corbusier also has a small niche in the history of modern painting as co-founder (with Ozenfant) of Purism in 1918. Up to 1929 he painted only still life, but from that time he occasionally introduced the human figure into his compositions. He retained the Purist dislike of ornament for its own sake, but his work became more dynamic, imaginative, and lyrical, though still restrained by his horror of any form of excess. He adopted the pseudonym Le Corbusier in 1920, but continued to sign his paintings ‘Jeanneret’; the pseudonym derives from the name of one of his grandparents and is also a pun on his facial resemblance to a raven (French corbeau). Apart from architecture and paintings, his enormous output included drawings, book illustrations, lithographs, tapestry designs, furniture, and numerous books, pamphlets, and articles.

Le Corbusier_London V&A_15Nov2008

His plan drawing for a stadium.

It seems like what my little brother did...It remind me that doesn't matter how beautiful is the plan drawing but how useful it is! I'm not a artist but a designer!


A way to present the session plan.